Students in Grades 3+ can more readily develop an understanding of multiplication when they see it visually. For example, they can picture a marching band arranged in equal rows or chairs set up evenly in an auditorium. In both cases, they are visualizing rows and columns. An arrangement of objects, pictures, or numbers in rows and columns is called an array. Arrays are useful representations of multiplication concepts (among other ideas in mathematics).
This array has 4 rows and 3 columns. It can also be described as a 4 by 3 array. The word "by" is often represented with a multiplication cross: 4 × 3.

This array has 5 rows and 4 columns. It is a 5 by 4 array.

Notice that the rows in each array are equal in length. Think of the rows as equal groups. Your students should have already used equal groups to multiply (2.OA.C.4).

If you arrange the equal groups in rows, you form an array.

When you show students the connection between equal groups and arrays, students can visualize how to use arrays to multiply. They will use arrays again later to divide.
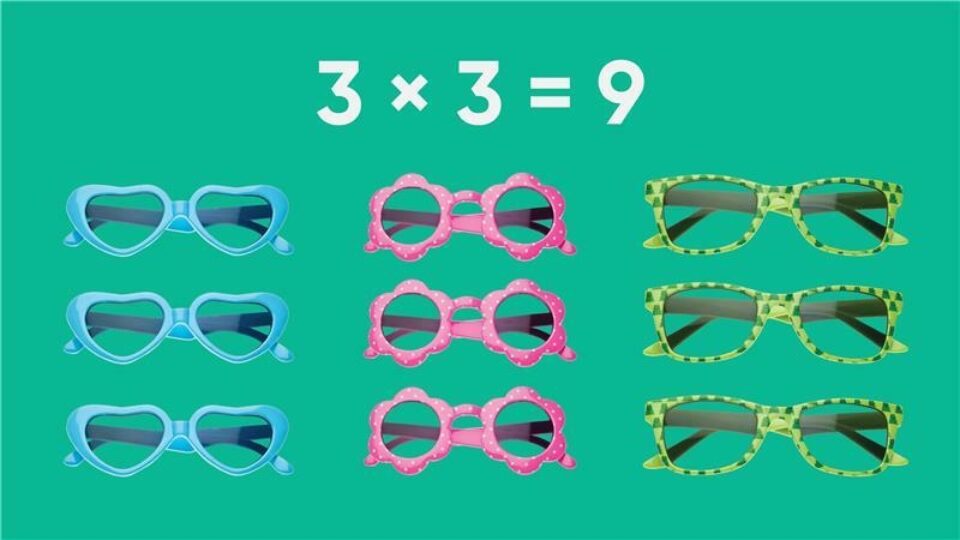
Look at the multiplication sentence that describes the array below. The numbers in multiplication sentences have special names.

The numbers that are multiplied are called factors. The result of multiplying the factors is called the product.
Now look at what happens to the factors and product in the multiplication sentence when the array is turned on its side.

The order of the factors changed, but the product stayed the same. This is in fact always the case and a feature that has piqued the interest of mathematicians for centuries. You can change the order of factors, but the product stays the same, a mathematical property known as the Commutative Property of Multiplication. Students should be familiar with the commutative property because they have already seen it applied to addition (1.OA.B.3).
Help students realize that by applying the commutative property, they know twice as many multiplication facts. For example, if they know 8 × 5 = 40, then they also know 5 × 8 = 40.
***
Looking for more free math lessons and activities for elementary school students? Be sure to explore our Free Teaching Resources portal.
Get our FREE guide "Optimizing the Math Classroom: 6 Best Practices."

















