
Numbers and the World Around Us
Is there anything interesting about the number 1,729 to you? If you want to partake in the thought experiment or provide it to your students, truly pause for a minute. Do you notice anything special or unusual about the number? Have you encountered it before? Why or why not?
A famous story goes like this: the legendary mathematician G.H. Hardy rode a taxi numbered 1729 on his way to meet another legend, the Indian mathematician Srinivasa Ramanujan. Hardy commented that the number was “dull.” Ramanujan disagreed instantly: “No,” he replied. “It is a very interesting number; it is the smallest number expressible as the sum of two cubes in two different ways.”
He's right, you know:
\[1,729=1^3+12^3\]
\[1,729=9^3+10^3\]
There is no smaller number that can be written as the sum of two different cubes like that. And it seems like pure magic that Ramanujan would know that immediately! To some extent, it is. He must have devoted thousands of hours to thinking about numbers in order to readily know this property, a devotion that to many of us might as well be magic.
However, this property—and Ramanujan’s thinking—can be unraveled by examining deeply the relationships between numbers. In this case, a number theorist as skilled as Ramanujan is likely to know the cubes through 20 off the top of his head:
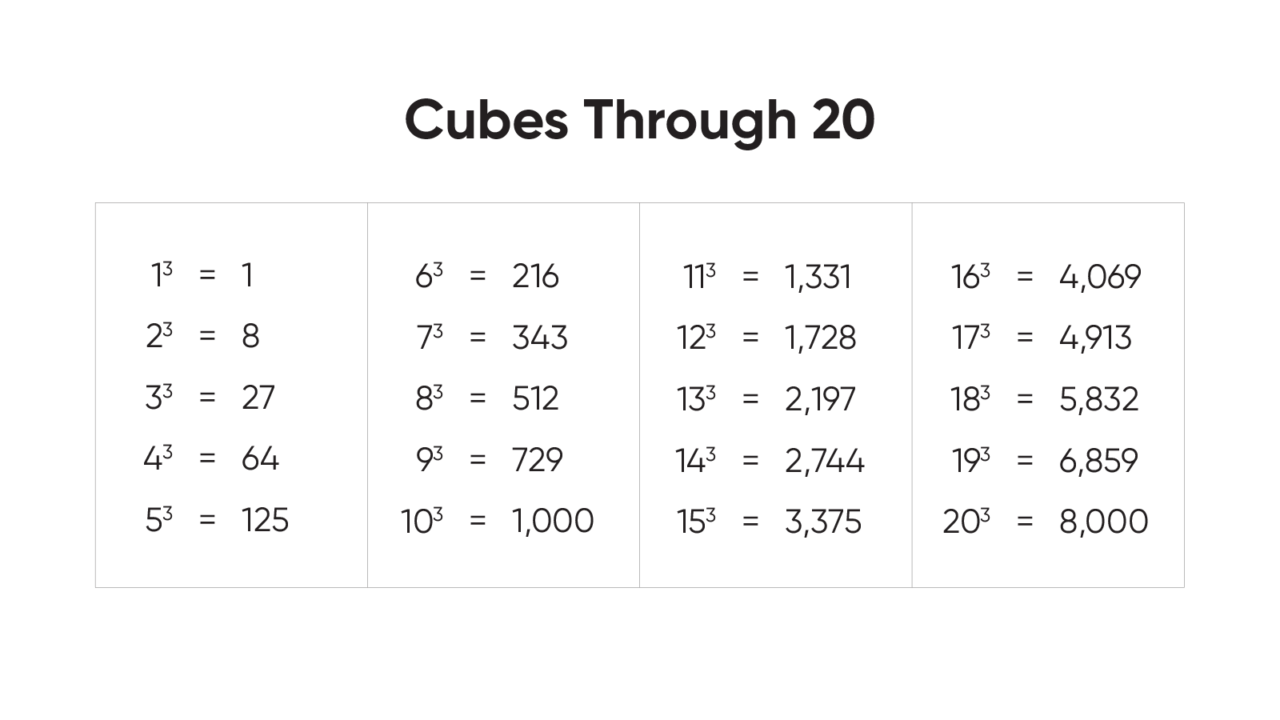
If you investigate this list long enough, you are bound to notice certain patterns and relationships. In fact, a problem that went unsolved for centuries is related to whether it was possible to add two cubes to get a third cube. (For more on that, see tip 7 in our post on helping your child with math at home.) It is not possible to find such a sum (try proving it!), but the cubes for 9, 10, and 12 come very close: 729 + 1,000 is almost 1,728.
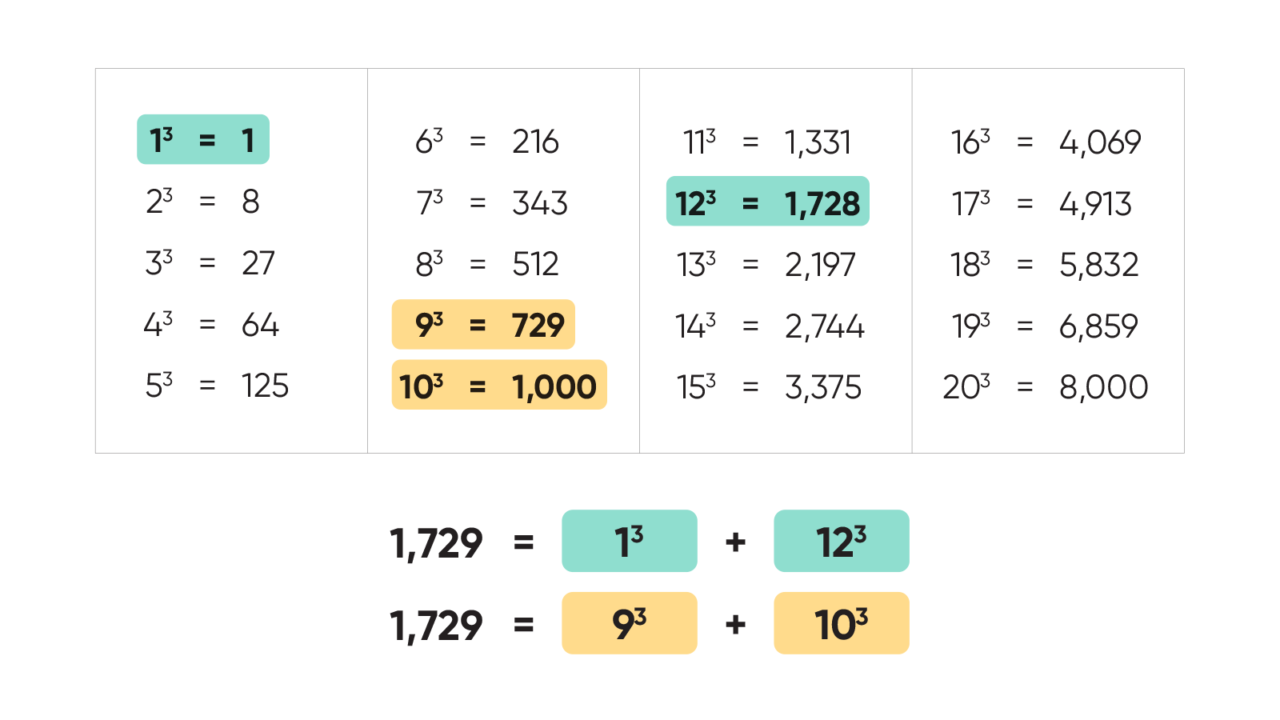
Suddenly, it’s not so shocking that Ramanujan recognized this property of 1,729:
\[1,729=1^3+12^3\]
\[1,729=9^3+10^3\]
There is a lesson here that goes beyond adding cubes. Taxicab numbers are just the start. We see numbers in game scores, sales figures, jersey numbers, years, and every discipline and context imaginable. These numbers do not exist in isolation, however; they form deeply interwoven patterns and can be analyzed, combined, and decomposed in endless ways. Imagine learning English without ever learning the patterns of prefixes and suffixes! By studying the relationships between numbers, your students will develop a richer number sense and grow to see relationships between numbers and life itself.
There are many ways to think about number relationships, and it can be difficult to show these different ideas to students. The Shaped archives have plenty of articles, activities, and resources to help you study them along with your students. Take a look at what we have to offer and help your students understand the value of number relationships and the many forms it can take.
Factors and Divisibility
One of the most common ways to investigate the relationships between numbers is by thinking about factors. 5 is related to 45, for example, because 5 is a factor of 45. This is a deep concept rooted in the fundamental theorem of arithmetic: every integer greater than 1 can be represented uniquely as a product of prime factors. The number 45, for example, can be thought of identically as the product of 3 • 3 • 5, with the factors allowed in any order. The Shaped posts below can help you introduce ideas of multiplication, division, and factors in the classroom.
Multiple Representations
A classic mathematical puzzle is this: is \(0.\overline{9}\) less than 1? (\(0.\overline{9}\) refers to 0.999… repeating forever, and spoiler: no, the two numbers are equal!) An aspect of mathematics that can be rich and counterintuitive is how one number can be represented in many ways. Being able to navigate the relationships between different representations, such as fractions, decimals, and percent or standard vs. scientific notation, helps with abstracting the concept of number and can help solve math problems of all types. We explore multiple representations of number in more detail across many posts:
Operations
Numbers and operations are frequently grouped together as a single subdiscipline of mathematics. The fact is that operations are a straightforward way to relate two numbers to each other: add them, subtract them, multiply them, divide them, and so on. The idea behind operations may be straightforward, but teaching them can be very challenging. Consider the flexibility required among all four operations when trying to describe how to split 100 objects among 6 people.
As students progress in their math education, they learn about the endless ways one can operate on numbers: exponents, absolute value, matrices, and all sorts of functions and rules. Consider these posts designed to help students with operations:
Expressions and Equations
What happens when multiple numbers are scrunched up into a single mathematical object? Sometimes, one or more of the numbers are expressed as unknown variables, as in the expression 7xy2. Other times, there is an equals sign, indicating that the numbers are in some way dependent on one another, for example in the equation 7 + 3 = x + 2. Being able to rewrite expressions and solve equations is a skill that is used and honed throughout all levels of mathematics.
Take a look at some of the articles and lessons we have to help your students get practice with working through mathematical expressions and equations.
Visual Models
One way to build students’ mental model for the relationship between numbers is to visualize them on a coordinate plane. Students can locate the numbers 3 and 7 on the coordinate plane and draw the point (3, 7). While graphing is clear and pervasive throughout math class, it only scratches the surface of ways to visualize mathematics.
There are many ways to illustrate number relationships, for example using data representations (how would you compare 35% and 38%?), fraction models (how would you compare 3 and 7, when 3 is a part and 7 is the whole?), or base ten models (how would you compare 100, 30, and 7 to the number 137?). Here are blog posts to help make the relationships between numbers visual for you and your students.
- Teaching with Math Place-Value Charts
- Teaching x- and y-Axis Graphing on Coordinate Grids
- Teaching Quadrant Numbers on a Graph
- Visualization: The Fourth Leg of Concrete–Pictorial–Abstract
- Why Visualization Is So Important in Your Math Classroom
- Teaching Math Through Art from the Metropolitan Museum of Art
Practicing the Relationship Between Numbers
This article walks through many of the way that students can develop mastery on the relationship between numbers. Ultimately, feeling comfortable with thinking about and manipulating numbers requires practice. And practice on doing more than just completing a standard algorithm!
When implementing the ideas or lessons within this article, the ultimate goal is to foster a sense of curiosity about numbers in kids that will last them a lifetime. Seek informal practice, such as asking students what they notice and wonder about numbers whenever they come up, even outside of math class. As students develop background knowledge in math and see numbers in new and different ways, who knows...maybe they will be the ones one day calling attention to interesting taxi numbers?
***
Looking for a math curriculum that will grow student confidence in mathematics and help learners learn the relationship between numbers? Explore our core math solutions HMH Into Math for Grades K–8 and HMH Into AGA for Grades 8–12.
Get our FREE guide "Optimizing the Math Classroom: 6 Best Practices."
Related Reading

Ms. Madge Alexandre, Miami-Dade reading teacher and Read 180 user















